Learning Objectives
Learning Objectives
By the end of this section, you will be able to do the following:
- Calculate the energy stored in a charged capacitor and the capacitance of a capacitor
- Explain the properties of capacitors and dielectrics
| capacitor | dielectric |
Capacitors
Capacitors
Consider again the X-ray tube discussed in the previous sample problem. How can a uniform electric field be produced? A single positive charge produces an electric field that points away from it, as in Figure 18.18. This field is not uniform, because the space between the lines increases as you move away from the charge. However, if we combine a positive and a negative charge, we obtain the electric field shown in Figure 18.20(a). Notice that, between the charges, the electric field lines are more equally spaced.
What happens if we place, say, five positive charges in a line across from five negative charges, as in Figure 18.29? Now the region between the lines of charge contains a fairly uniform electric field.
We can extend this idea even further and into two dimensions by placing two metallic plates face to face and charging one with positive charge and the other with an equal magnitude of negative charge. This can be done by connecting one plate to the positive terminal of a battery and the other plate to the negative terminal, as shown in Figure 18.30. The electric field between these charged plates will be extremely uniform.
Let’s think about the work required to charge these plates. Before the plates are connected to the battery, they are neutral—that is, they have zero net charge. Placing the first positive charge on the left plate and the first negative charge on the right plate requires very little work, because the plates are neutral, so no opposing charges are present. Now consider placing a second positive charge on the left plate and a second negative charge on the right plate. Because the first two charges repel the new arrivals, a force must be applied to the two new charges over a distance to put them on the plates. This is the definition of work, which means that, compared with the first pair, more work is required to put the second pair of charges on the plates. To place the third positive and negative charges on the plates requires yet more work, and so on. Where does this work come from? The battery! Its chemical potential energy is converted into the work required to separate the positive and negative charges.
Although the battery does work, this work remains within the battery-plate system. Therefore, conservation of energy tells us that, if the potential energy of the battery decreases to separate charges, the energy of another part of the system must increase by the same amount. In fact, the energy from the battery is stored in the electric field between the plates. This idea is analogous to considering that the potential energy of a raised hammer is stored in Earth’s gravitational field. If the gravitational field were to disappear, the hammer would have no potential energy. Likewise, if no electric field existed between the plates, no energy would be stored between them.
If we now disconnect the plates from the battery, they will hold the energy. We could connect the plates to a lightbulb, for example, and the lightbulb would light up until this energy was used up. These plates thus have the capacity to store energy. For this reason, an arrangement such as this is called a capacitor. A capacitor is an arrangement of objects that, by virtue of their geometry, can store energy an electric field.
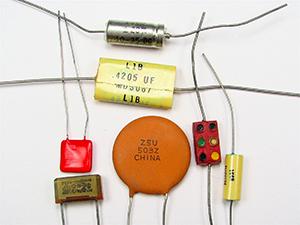
Various real capacitors are shown in Figure 18.31. They are usually made from conducting plates or sheets that are separated by an insulating material. They can be flat or rolled up or have other geometries.
The capacity of a capacitor is defined by its capacitance C, which is given by
where Q is the magnitude of the charge on each capacitor plate, and V is the potential difference in going from the negative plate to the positive plate. This means that both Q and V are always positive, so the capacitance is always positive. We can see from the equation for capacitance that the units of capacitance are C/V, which are called farads (F) after the nineteenth-century English physicist Michael Faraday.
The equation makes sense: A parallel-plate capacitor (like the one shown in Figure 18.30) the size of a football field could hold a lot of charge without requiring too much work per unit charge to push the charge into the capacitor. Thus, Q would be large, and V would be small, so the capacitance C would be very large. Squeezing the same charge into a capacitor the size of a fingernail would require much more work, so V would be very large, and the capacitance would be much smaller.
Although the equation makes it seem that capacitance depends on voltage, in fact it does not. For a given capacitor, the ratio of the charge stored in the capacitor to the voltage difference between the plates of the capacitor always remains the same. Capacitance is determined by the geometry of the capacitor and the materials that it is made from. For a parallel-plate capacitor with nothing between its plates, the capacitance is given by
where A is the area of the plates of the capacitor and d is their separation. We use instead of C, because the capacitor has nothing between its plates (in the next section, we’ll see what happens when this is not the case). The constant read epsilon zero is called the permittivity of free space, and its value is
Coming back to the energy stored in a capacitor, we can ask exactly how much energy a capacitor stores. If a capacitor is charged by putting a voltage V across it for example, by connecting it to a battery with voltage V—the electrical potential energy stored in the capacitor is
Notice that the form of this equation is similar to that for kinetic energy, .
Watch Physics
Where does Capacitance Come From?
This video shows how capacitance is defined and why it depends only on the geometric properties of the capacitor, not on voltage or charge stored. In so doing, it provides a good review of the concepts of work and electric potential.
If you increase the distance between the plates of a capacitor, how does the capacitance change?
- Doubling the distance between capacitor plates will reduce the capacitance four fold.
- Doubling the distance between capacitor plates will reduce the capacitance two fold.
- Doubling the distance between capacitor plates will increase the capacitance two times.
- Doubling the distance between capacitor plates will increase the capacitance four times.
Virtual Physics
Charge your Capacitor
For this simulation, choose the tab labeled Introduction at the top left of the screen. You are presented with a parallel-plate capacitor connected to a variable-voltage battery. The battery is initially at zero volts, so no charge is on the capacitor. Slide the battery slider up and down to change the battery voltage, and observe the charges that accumulate on the plates. Display the capacitance, top-plate charge, and stored energy as you vary the battery voltage. You can also display the electric-field lines in the capacitor. Finally, probe the voltage between different points in this circuit with the help of the voltmeter, and probe the electric field in the capacitor with the help of the electric-field detector.
True or false— In a capacitor, the stored energy is always positive, regardless of whether the top plate is charged with negative or positive charge.
- false
- true
Worked Example
Capacitance and Charge Stored in a Parallel Plate Capacitor
(a) What is the capacitance of a parallel-plate capacitor with metal plates, each of area 1.00 m2, separated by 0.0010 m? (b) What charge is stored in this capacitor if a voltage of 3.00 × 103 V is applied to it?
STRATEGY FOR (A)
Use the equation .
Entering the given values into this equation for the capacitance of a parallel-plate capacitor yields
This small value for the capacitance indicates how difficult it is to make a device with a large capacitance. Special techniques help, such as using very-large-area thin foils placed close together or using a dielectric (to be discussed below).
STRATEGY FOR (B)
Knowing C, find the charge stored by solving the equation , for the charge Q.
The charge Q on the capacitor is
This charge is only slightly greater than typical static electricity charges. More charge could be stored by using a dielectric between the capacitor plates.
Worked Example
What battery is needed to charge a capacitor?
Your friend provides you with a capacitor. To store on this capacitor, what voltage battery should you buy?
STRATEGY
Use the equation to find the voltage needed to charge the capacitor.
Solving for the voltage gives . Inserting and gives
Such a battery should be easy to procure. There is still a question of whether the battery contains enough energy to provide the desired charge. The equation allows us to calculate the required energy.
A typical commercial battery can easily provide this much energy.
Practice Problems
Practice Problems
What is the voltage on a 35 μF with 25 nC of charge?
- 8.75 × 10−13 V
- 0.71 × 10−3 V
- 1.4 × 10−3 V
- 1.4 × 103 V
Which voltage is across a 100 μF capacitor that stores 10 J of energy?
- −4.5 × 102 V
- 4.5 × 102 V
- ±4.5 × 102 V
- ±9 × 102 V
Dielectrics
Dielectrics
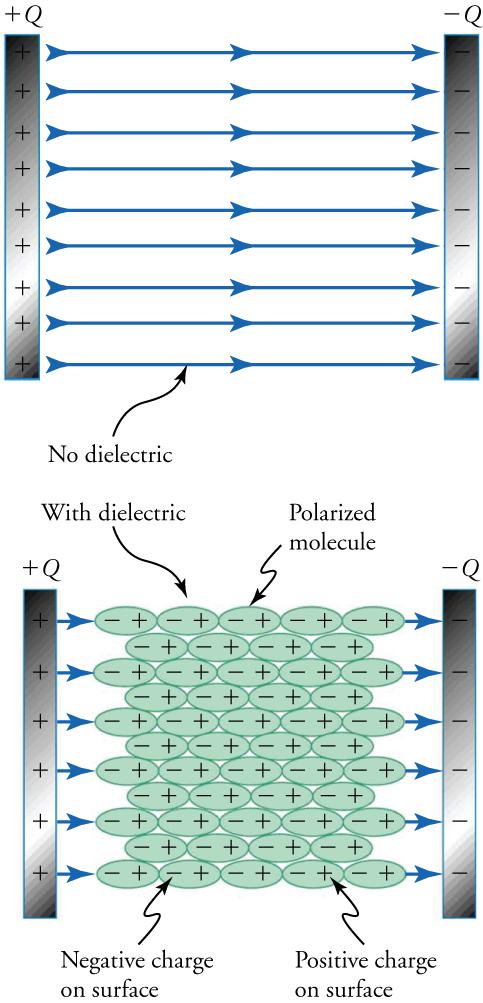
Before working through some sample problems, let’s look at what happens if we put an insulating material between the plates of a capacitor that has been charged and then disconnected from the charging battery, as illustrated in Figure 18.33. Because the material is insulating, the charge cannot move through it from one plate to the other, so the charge Q on the capacitor does not change. An electric field exists between the plates of a charged capacitor, so the insulating material becomes polarized, as shown in the lower part of the figure. An electrically insulating material that becomes polarized in an electric field is called a dielectric.
Figure 18.33 shows that the negative charge in the molecules in the material shifts to the left, toward the positive charge of the capacitor. This shift is due to the electric field, which applies a force to the left on the electrons in the molecules of the dielectric. The right sides of the molecules are now missing a bit of negative charge, so their net charge is positive.
All electrically insulating materials are dielectrics, but some are better dielectrics than others. A good dielectric is one whose molecules allow their electrons to shift strongly in an electric field. In other words, an electric field pulls their electrons a fair bit away from their atom, but they do not escape completely from their atom (which is why they are insulators).
Figure 18.34 shows a macroscopic view of a dielectric in a charged capacitor. Notice that the electric-field lines in the capacitor with the dielectric are spaced farther apart than the electric-field lines in the capacitor with no dielectric. This means that the electric field in the dielectric is weaker, so it stores less electrical potential energy than the electric field in the capacitor with no dielectric.
Where has this energy gone? In fact, the molecules in the dielectric act like tiny springs, and the energy in the electric field goes into stretching these springs. With the electric field thus weakened, the voltage difference between the two sides of the capacitor is smaller, so it becomes easier to put more charge on the capacitor. Placing a dielectric in a capacitor before charging it therefore allows more charge and potential energy to be stored in the capacitor. A parallel plate with a dielectric has a capacitance of
where (kappa) is a dimensionless constant called the dielectric constant. Because is greater than 1 for dielectrics, the capacitance increases when a dielectric is placed between the capacitor plates. The dielectric constant of several materials is shown in Table 18.1.
| Material | Dielectric Constant () |
|---|---|
| Vacuum | 1.00000 |
| Air | 1.00059 |
| Fused quartz | 3.78 |
| Neoprene rubber | 6.7 |
| Nylon | 3.4 |
| Paper | 3.7 |
| Polystyrene | 2.56 |
| Pyrex glass | 5.6 |
| Silicon oil | 2.5 |
| Strontium titanate | 233 |
| Teflon | 2.1 |
| Water | 80 |
Worked Example
Capacitor for Camera Flash
A typical flash for a point-and-shoot camera uses a capacitor of about . (a) If the potential difference between the capacitor plates is 100 V—that is, 100 V is placed “across the capacitor,” how much energy is stored in the capacitor? (b) If the dielectric used in the capacitor were a 0.010-mm-thick sheet of nylon, what would be the surface area of the capacitor plates?
STRATEGY FOR (A)
Given that and , we can use the equation , to find the electric potential energy stored in the capacitor.
Inserting the given quantities into gives
This is enough energy to lift a 1-kg ball about 1 m up from the ground. The flash lasts for about 0.001 s, so the power delivered by the capacitor during this brief time is . Considering that a car engine delivers about 100 kW of power, this is not bad for a little capacitor!
STRATEGY FOR (B)
Because the capacitor plates are in contact with the dielectric, we know that the spacing between the capacitor plates is . From the previous table, the dielectric constant of nylon is . We can now use the equation to find the area A of the capacitor.
Solving the equation for the area A and inserting the known quantities gives
This is much too large an area to roll into a capacitor small enough to fit in a handheld camera. This is why these capacitors don’t use simple dielectrics but a more advanced technology to obtain a high capacitance.
Practice Problems
Practice Problems
With 12 V across a capacitor, it accepts 10 mC of charge. What is its capacitance?
- 0.83 μF
- 83 μF
- 120 μF
- 830 μF
A parallel-plate capacitor has an area of 10 cm2 and the plates are separated by 100 μm . If the capacitor contains paper between the plates, what is its capacitance?
- 3.3 × 10−10 F
- 3.3 × 10−8 F
- 3.3 × 10−6 F
- 3.3 × 10−4 F
Check Your Understanding
Check Your Understanding
- The capacitance will remain same.
- The capacitance will double.
- The capacitance will increase four times.
- The capacitance will increase eight times.
- It will increase by a factor of two.
- It will increase by a factor of four.
- It will increase by a factor of six.
- It will increase by a factor of eight.